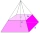
Pyramid cut
We cut the regular square pyramid with a parallel plane to the two parts (see figure). The volume of the smaller pyramid is 20% of the volume of the original one. The bottom of the base of the smaller pyramid has an area of 10 cm2. Find the area of the original pyramid.
Your answer:
Your answer:
Tips for related online calculators
Our percentage calculator will help you quickly and easily solve a variety of common percentage-related problems.
Tip: Our volume units converter will help you convert volume units.
See also our trigonometric triangle calculator.
Tip: Our volume units converter will help you convert volume units.
See also our trigonometric triangle calculator.
You need to know the following knowledge to solve this word math problem:
geometryalgebraarithmeticsolid geometryplanimetrybasic operations and conceptsUnits of physical quantitiesGrade of the word problem
We encourage you to watch this tutorial video on this math problem: video1
Related math problems and questions:
- Pyramid volume ratio
A regular quadrilateral pyramid with base edge length a = 15 cm and height v = 21 cm is given. We draw two planes parallel to the base, dividing the height of the pyramid into three equal parts. Calculate the ratio of the volumes of the 3 bodies created. - Pyramid cutting calculation
The regular quadrilateral pyramid has a height of 40 cm and a base side of 21 cm. Cut the needle at half the height. How much will both parts have? - 2x cone
A circular cone of height 36 cm is cut by a plane parallel to the base, dividing it into two smaller cones of equal volume. Calculate the height of the smaller cone. - Quadrangular pyramid
Calculate the surface area and volume of a regular quadrilateral pyramid: sides of bases (bottom, top): a1 = 18 cm, a2 = 6 cm angle α = 60 ° (Angle α is the angle between the sidewall and the base plane.) S =? , V =? - Right circular cone
The volume of a right circular cone is 5 liters. The cone is divided by a plane parallel to the base, one-third down from the vertex to the base. Calculate the volume of these two parts of the cone. - Digging a pit
The pit has the shape of a regular quadrilateral truncated pyramid. The edges of the bases are 14 m and 10 m long. The sidewalls form an angle of 135° with a smaller base. Find how many m³ of soil were excavated when digging the pit. - Quadrilateral pyramid
Calculate the volume of a regular quadrilateral pyramid, which has the size of the base edge a = 12 cm and a height of 11 cm.
