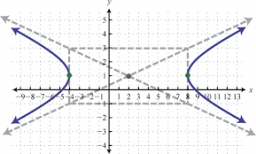
Hyperbola equation
Find the hyperbola equation with the center of S [0; 0], passing through the points:
A [5; 3] B [8; -10]
A [5; 3] B [8; -10]
Correct answer:
Tips for related online calculators
Are you looking for help with calculating roots of a quadratic equation?
Do you have a linear equation or system of equations and looking for its solution? Or do you have a quadratic equation?
Do you have a linear equation or system of equations and looking for its solution? Or do you have a quadratic equation?
You need to know the following knowledge to solve this word math problem:
We encourage you to watch this tutorial video on this math problem: video1
Related math problems and questions:
- Hyperbola
Find the equation of hyperbola that passes through the point M [30; 24] and has focal points at F1 [0; 4 sqrt 6], F2 [0; -4 sqrt 6]. - Calculate 6
Calculate the distance of point A[0, 2] from a line passing through points B[9, 5] and C[1, -1]. - Line
Straight-line passing through points A [-3; 22] and B [33; -2]. Determine the total number of points of the line in which both coordinates are positive integers. - Linear function
What is the equation of linear function passing through points: a) A (0,3), B (3,0) b) A (-2,-6), B (3,4) - Parabola
Find the equation of a parabola that contains the points at A[10; -5], B[18; -7], C[20; 0]. (use y = ax²+bx+c) - Equation 80525
Write the equation of the parabola that passes through the points: A[1,1] B[3,-1] C[1,2] - Coordinates 65224
The line PQ is determined by points with coordinates P = [- 2; 4] and Q = [4; 0]. What are the coordinates of the center S of the line segment PQ? - Direction vector
The line p is given by the point P [- 0,5; 1] and the direction vector s = (1,5; - 3) determines: A) value of parameter t for points X [- 1,5; 3], Y [1; - 2] lines p B) whether the points R [0,5; - 1], S [1,5; 3] lies on the line p C) parametric equations - Circle
The circle is given by center on S[-7; 10] and maximum chord 13 long. How many intersect points have a circle with the coordinate axes? - Center
In the ABC triangle is point D[1,-2,6], which is the center of the |BC|, and point G[8,1,-3], which is the center of gravity of the triangle. Find the coordinates of the vertex A[x,y,z]. - MO circles
Juro built the ABCD square with a 12 cm side. In this square, he scattered a quarter circle with a center at point B passing through point A and a semicircle l with a center at the center of the BC side and passed point B. He would still build a circle th - Sphere equation
Obtain the equation of a sphere. Its center is on the line 3x+2z=0=4x-5y and passes through the points (0,-2,-4) and (2,-1,1). - Rectangular 75334
In the rectangular coordinate system, find the images of points A[-3; 2] and B[4; -5] in central symmetry according to point O[0; 0]. A. A'[3; 2], B'l-4; -5] C. A'[-3; -2], B'[4; 5] B. A'[-3; -2], B'[-4; 5] D. A'[3; -2], B'[-4; 5] - Center of gravity
The mass points are distributed in space as specified by coordinates and weight. Find the center of gravity of the mass points system: A1 [1; -20; 3] m1 = 46 kg A2 [-20; 2; 9] m2 = 81 kg A3 [9; -2; -1 - On line
On line p: x = 4 + t, y = 3 + 2t, t is R, find point C, which has the same distance from points A [1,2] and B [-1,0]. - Touch x-axis
Find the equations of circles that pass through points A (-2; 4) and B (0; 2) and touch the x-axis. - Circle
Write the equation of a circle that passes through the point [0,6] and touches the X-axis point [5,0]: (x-x_S)²+(y-y_S)²=r²
