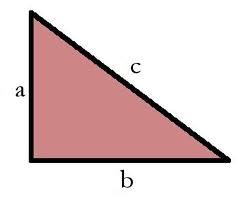
RT and circles
Solve the right triangle if the radius of the inscribed circle is r=9 and the radius of the circumscribed circle is R=23.
Correct answer:
Tips for related online calculators
Are you looking for help with calculating roots of a quadratic equation?
See also our right triangle calculator.
See also our trigonometric triangle calculator.
See also our right triangle calculator.
See also our trigonometric triangle calculator.
You need to know the following knowledge to solve this word math problem:
Grade of the word problem:
Related math problems and questions:
- Regular n-gon
Which regular polygon has a radius of circumscribed circle r = 10 cm and the radius of inscribed circle p = 9.962 cm? - Annular area
The square with side a = 1 is inscribed and circumscribed by circles. Find the annular area. - Annulus from triangle
Calculate the area of the area bounded by a circle circumscribed and a circle inscribed by a triangle with sides a = 25mm, b = 29mm, c = 36mm. - Diagonals of pentagon
Calculate the diagonal length of the regular pentagon: a) inscribed in a circle of radius 12dm; b) a circumscribed circle with a radius of 12dm.
- Dodecagon
Find the area of a regular dodecagon (n=12) if the radius of the circumscribed circle is 5 cm. - Circumscribed 22713
Calculate the circumference and the area of a regular ten-angle polygon if the radius of the circumscribed circle r = 20 cm. - Circumscribed 63824 In a regular decagon, the diameter of the circumscribed circle measures 10 cm. Determine the radius of the circle inscribed in this triangle.
