Pythagorean theorem - math word problems - page 54 of 73
Number of problems found: 1446
- Calculate 30971
Calculate the cone's surface and volume if its base diameter is 1 dm and the side length is 13 cm. - Pyramid-shaped 30191
Above the pavilion, with a square floor plan with side a = 12 m, is a pyramid-shaped roof with a height of 4.5 m. How many m² of sheet metal is needed to cover this roof? - Rotating 28001
There is a rotating cone: r = 6.8 cm s = 14.4 cm. Find the area of the cone surface S2, the height h, and the volume V. - Four-sided 7833
The tower has the shape of a regular four-sided pyramid with a base edge of 0.8 m. The height of the tower is 1.2 meters. How many square meters of sheet metal is needed for coverage if we count eight percent for joints and overlap? - Circular 4690
The cone shell with a base radius of 20 cm and a height of 50 cm unfolds into a circular cutout. How big is the center angle of this cutout? - Calculate 82409
The lamp shade should be formed by the shell of a cone with a base diameter of 48 cm and a side of 32 cm. Calculate how much material will be needed to make it, assuming 8% waste - Equilateral shell
The glass weight has the shape of a regular four-sided pyramid with a base edge of 10 cm. The shell walls are equilateral triangles. What is the weight in grams of the paperweight if the density of the glass is 2500 kg/m³? - A Pile of salt
A Pile of salt has been stored in the shape of a cone. Mr. Terwilliker knows that the pile is 20 feet tall and 102 feet in circumference at the base. What area of the conical tarpaulin (a large sheet of material) is needed to cover the pile? - Total area
Calculate the total area (surface and bases) of a prism whose base is a rhombus with 12cm and 18cm diagonals and whose prism height is 10 cm. - The bus stop
The bus stop waiting room has the shape of a regular quadrilateral pyramid 4 m high with a 5 m base edge. Calculate how much m² roofing is required to cover the sheathing of three walls, taking 40% of the additional coverage. - Hexagon cut pyramid
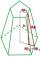Calculate the volume of a regular 6-sided cut pyramid if the bottom edge is 30 cm, the top edge is 12 cm, and the side edge length is 41 cm. - Four sided prism
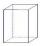Calculate the volume and surface area of a regular quadrangular prism whose height is 28.6cm, and the diagonal body forms a 50-degree angle with the base plane. - The glass
The glass has the shape of a cylinder with an inner diameter of 12 cm, and the height from the bottom is 16 cm. The cut skewer can be inserted diagonally into the glass so it does not protrude beyond the edge. What is the largest possible length of the cu - Tent
A pyramid-shaped tent has a base square with a side length of 2 m and a height of 1.7 m. How many meters of canvas is needed to make it if we should add 10% for waste? - Diameter 33361
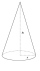The roof of the castle tower has the shape of a cone with a base diameter of 12 m and a height of 8 m. How many euros will we pay to cover the roof if 1m of square roofing costs 3.5 euros? - Consumption 15663
The cone-shaped sheet metal roof has a base diameter of 80 cm and a height of 60 cm. If 1 kg of paint is consumed per 6 m² of sheet metal, calculate the paint consumption for painting this roof. - Three-walled 8272
I have only entered the height of a regular three-walled pyramid h = 10 cm. How do I calculate its edge length? - Centimeters 6596
The jewelry box is in the shape of a four-sided prism with the base of an isosceles trapezoid with sides a=15 centimeters, b is equal to 9 centimeters, c is equal to 10 centimeters, c is equal to 7 whole 4 centimeters. How much fabric is needed to cover a - Dimensions 39623
In the form of a pyramid with a square floor plan, the house's roof has dimensions of 12 x 12 m, at the highest point, a height of 2 m. How much roofing do we need to buy? Count on a 10% reserve. - Quadrangular prism Calculate the volume and surface area of a regular quadrangular prism 35 cm high and the base diagonal 22 cm.
Do you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
