Pythagorean theorem - math word problems - page 53 of 73
Number of problems found: 1446
- Dimensions of a fabric
How many m² of fabric is needed to make a tent of a regular 3-sided prism if it is necessary to count on a 2% reserve of fabric? Dimensions - 2m 1.6m and height 1.4m - Cube from sphere
What largest surface area (in cm²) can have a cube that we cut out of a sphere with a radius 26 cm? - Bottles of juice
How many 2-liter bottles of juice need to buy if you want to transfer the juice to 50 pitchers' rotary cone shape with a diameter of 24 cm and a base side length of 1.5 dm? - Prism
A right-angled prism, whose base is a right triangle with leg a = 3 cm and hypotenuse c = 6 cm, has the same volume as a cube with an edge length of 1 dm. a) Find the height of the prism b) Calculate the surface of the prism c) What percentage of the cube - Cone A2V
The cone's surface in the plane is a circular arc with a central angle of 126° and an area of 415 cm². Calculate the volume of a cone. - Side wall planes
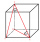Find the volume and surface of a cuboid whose side c is 30 cm long and whose body diagonal forms angles of 24°20' and 45°30' with the planes of the side walls. - Quadrilateral 33143
The roof of the prefabricated holiday cottage has the shape of a regular quadrilateral pyramid with a length of the base edge of 8 meters and a height of 9 m. How many square meters of cardboard are needed to cover the roof? - Administrator 23801
The administrator of the castle is trying to estimate how many square meters of sheet metal will be needed for the new roof of the tower. The roof has the shape of a cone. The castle administrator knows that the tower's diameter is 4.6 meters and its heig - Quadrilateral 8120
If the pyramid's height is 4 cm and the base area is 16 cm2, include the side edge length of a regular quadrilateral pyramid. - Dimensions - crate
A wooden crate with dimensions d=3m, e=4m, and f=3m was placed in a transport container with dimensions a=10 m, b=4m, and c=3m. What is the maximum length of a straight, rigid rod of negligible diameter that can still be placed in the container in this si - Metal balls
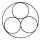Four metal balls with a diameter of 5 cm are placed in a measuring cylinder with an inner diameter of 10 cm. What is the smallest water volume to be poured into the cylinder so that all balls are below the water level? - Convex lens
The convex lens consists of two spherical segments (dimensions given in mm). Calculate its weight if the density of the glass is 2.5 g/cm³. Dimensions: 60mm in length and width of the upper part 5mm, the width of the lower part 8mm - How many
How many m² of copper sheet is needed to replace the roof of a conical tower with a diameter of 13 meters and a height of 24 meters if we count 8% of the material for bending and waste? - Triangular prism,
The regular triangular prism, whose edges are identical, has a surface of 2514 cm² (square). Find the volume of this body in cm³ (l). - The pyramid 4s
The pyramid with a rectangular base measuring 6 dm and 8 dm has a side edge of a length of 13 dm. Calculate the surface area and volume of this pyramid. - Hexagonal pyramid
Calculate the surface area of a regular hexagonal pyramid with a base inscribed in a circle with a radius of 8 cm and a height of 20 cm. - Vertical prism The base of the vertical prism is a right triangle with leg a = 5 cm and a hypotenuse c = 13 cm. The height of the prism is equal to the circumference of the base. Calculate the surface area and volume of the prism.
- Sphere cuts
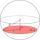At what distance from the center does the sphere intersect with the radius R = 46 plane if the cut area and area of the main sphere circle are in ratio 2/5? - Sandpile
Auto sprinkled with sand to an approximately conical shape. Workers wanted to determine the volume (amount of sand) and, therefore, measure the base's circumference and the length of both sides of the cone (over the top). What is the sand cone's volume if - Quadrilateral 40551
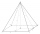Find the volume and surface area of a regular quadrilateral pyramid ABCDV if its leading edge has a length a = 10 cm and a body height h = 12 cm.
Do you have unsolved math question and you need help? Ask a question, and we will try to solve it. We solve math question.
