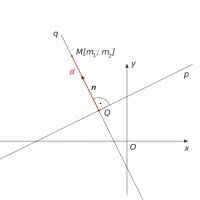
Parametric form
Calculate the distance of point A [2,1] from the line p:
X = -1 + 3 t
Y = 5-4 t
Line p has a parametric form of the line equation.
X = -1 + 3 t
Y = 5-4 t
Line p has a parametric form of the line equation.
Correct answer:
Tips for related online calculators
Our vector sum calculator can add two vectors given by their magnitudes and by included angle.
Do you want to convert length units?
See also our right triangle calculator.
See also our trigonometric triangle calculator.
Do you want to convert length units?
See also our right triangle calculator.
See also our trigonometric triangle calculator.
You need to know the following knowledge to solve this word math problem:
Units of physical quantities:
Grade of the word problem:
Related math problems and questions:
- On line
On line p: x = 4 + t, y = 3 + 2t, t is R, find point C, which has the same distance from points A [1,2] and B [-1,0]. - General line equations
In all examples, write the GENERAL EQUATION OF a line that is given in some way. A) the line is given parametrically: x = - 4 + 2p, y = 2 - 3p B) the slope form gives the line: y = 3x - 1 C) the line is given by two points: A [3; -3], B [-5; 2] D) the lin - Perpendicular projection Determine the distance of a point B[1, -3] from the perpendicular projection of a point A[3, -2] on a straight line 2 x + y + 1 = 0.
- Parametric equation Point A [6; -2]. Point B = [-3; 1] Write the parametric equation of the line BA so that t belongs to the closed interval < 0;3 >
- Calculate 8
Calculate the coordinates of point B axially symmetrical with point A[-1, -3] along a straight line p : x + y - 2 = 0. - Direction vector The line p is given by the point P [- 0,5; 1] and the direction vector s = (1,5; - 3) determines: A) value of parameter t for points X [- 1,5; 3], Y [1; - 2] lines p B) whether the points R [0,5; - 1], S [1,5; 3] lies on the line p C) parametric equations
- Parametric equations
Write the parametric equations of height hc in triangle ABC: A = [5; 6], B = [- 2; 4], C = [6; -1] - Determine 82478
Determine the equation of the parabola that has the point F = [3,2] as its focus and the line x+y+1=0 as its shift line. - Parametric 82072
Convert the parametric expression of the straight line to a general equation. x=3-5t y=-4+10t - Tangents to ellipse
Find the magnitude of the angle at which the ellipse x² + 5 y² = 5 is visible from the point P[5, 1]. - Find the
Find the image A' of point A [1,2] in axial symmetry with the axis p: x = -1 + 3t, y = -2 + t (t = are real number) - Center of line segment
Calculate the distance of point X [1,3] from the center of the line segment x = 2-6t, y = 1-4t; t is from interval <0,1>. - Line
Write an equation of a line parallel to To 9x + 3y = 8 That Passes Through The Point (-1, -4). Write in the form ax+by=c. - Coefficient 81704
In the equation of the line p: ax-2y+1=0, determine the coefficient a so that the line p: a) it formed an angle of 120° with the positive direction of the x-axis, b) passed through point A[3,-2], c) was parallel to the x-axis, d) had a direction of k = 4. - Slope form
Find the equation of a line given the point X(8, 1) and slope -2.8. Arrange your answer in the form y = ax + b, where a and b are the constants. - Calculate 6
Calculate the distance of point A[0, 2] from a line passing through points B[9, 5] and C[1, -1]. - Find the 5
Find the equation of the circle with the center at (1,20), which touches the line 8x+5y-19=0
