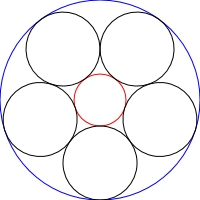
Annulus
Two concentric circles with radii 1 and 9 surround the annular circle. This ring is inscribed with n circles that do not overlap. Determine the highest possible value of n.
Correct answer:
Tips for related online calculators
See also our trigonometric triangle calculator.
You need to know the following knowledge to solve this word math problem:
Units of physical quantities:
Grade of the word problem:
We encourage you to watch this tutorial video on this math problem: video1
Related math problems and questions:
- Annular area
The square with side a = 1 is inscribed and circumscribed by circles. Find the annular area. - Concentric 18343
Construct three concentric circles k, l, m with center at point S and with radii 2cm, 3cm, and 40mm - Concentric circles
In the circle with diameter, 13 cm is constructed chord 1 cm long. Calculate the radius of a concentric circle that touches this chord. - Circular ring
A square with an area of 16 centimeters is inscribed circle k1 and described to circle k2. Calculate the area of the circular ring, which circles k1, and k2 form.
- Centimeters 6220
A pizza in the shape of a circle occupies an area of 94.985 square cm. What is the smallest integer diameter in centimeters that the plate on which we want to place this pizza must have so that the plates do not overlap - Right-angled 78394
A right-angled triangle was inscribed in a circle with a diameter of 20 cm, whose hypotenuse is the circle's diameter and has the largest possible area. Calculate the area of this triangle. - Two annuluses
The area of the annular circle formed by two circles with a common center is 100 cm². The radius of the outer circle is equal to twice the radius of the inner circle. Determine the outside circle radius in centimeters.
