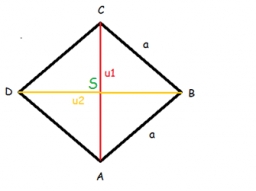
Diamond area from diagonals
In the diamond, ABCD is AB = 4 dm, and the diagonal length is 6.4 dm long. What is the area of the diamond?
Final Answer:
Tips for related online calculators
You need to know the following knowledge to solve this word math problem:
planimetricsGrade of the word problem
Related math problems and questions:
- The diagonals
The diagonals in the diamond ABCD are 6 cm and 8 cm long. What is the perimeter of this diamond? - Trapezoid - intersection of diagonals
In the ABCD trapezoid is AB = 8 cm long, trapezium height 6 cm, and distance of diagonals intersection from AB is 4 cm. Calculate the trapezoid area. - Trapezium diagonals
It is given trapezium ABCD with bases | AB | = 12 cm, |CD| = 8 cm. Point S is the intersection of the diagonals for which |AS| is 6 cm long. Calculate the length of the full diagonal AC. - Diagonals in diamons/rhombus
Rhombus ABCD has a side length AB = 4 cm and a length of one diagonal of 6.4 cm. Calculate the length of the other diagonal. - Circumference 26361
The ABCD diamond has a circumference of 72 cm. The longer diagonal of the animal with the line segment AB angle is 30 °. Calculate the area of the ABCD diamond. - Flowerbed 2540
The flowerbed has a diamond shape with side a = 35 dm. The longer diagonal is 56 dm long. Calculate the area of the flowerbed. - Diagonal intersect
Isosceles trapezoid ABCD with length bases | AB | = 6 cm, CD | = 4 cm is divided into four triangles by the diagonals intersecting at point S. How much of the area of the trapezoid are ABS and CDS triangles?
