Water channel
The cross-section of the water channel is a trapezoid. The bottom width is 19.7 m, the water surface width is 28.5 m, and the side walls have a slope of 67°30' and 61°15'. Calculate how much water flows through the channel in 5 minutes if the water flows at a rate of 0.3 m/s.
Correct answer:
Tips for related online calculators
The line slope calculator is helpful for basic calculations in analytic geometry. The coordinates of two points in the plane calculate slope, normal and parametric line equation(s), slope, directional angle, direction vector, the length of the segment, intersections of the coordinate axes, etc.
Tip: Our volume units converter will help you convert volume units.
Do you want to convert velocity (speed) units?
See also our right triangle calculator.
See also our trigonometric triangle calculator.
Tip: Our volume units converter will help you convert volume units.
Do you want to convert velocity (speed) units?
See also our right triangle calculator.
See also our trigonometric triangle calculator.
You need to know the following knowledge to solve this word math problem:
algebrasolid geometryplanimetricsgoniometry and trigonometryUnits of physical quantitiesGrade of the word problem
Related math problems and questions:
- Cross-section - trapezoid
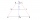The cross-section of the channel has the shape of a trapezoid. The bottom width is 2.25 m, and the depth is 5 m. The walls have a slope of 68°12' and 73°45'. Calculate the upper channel width. - Cross-sectional 18973
Calculate the cross-sectional area of the trapezoidal channel irrigation channel if its upper width is 7.9 m, its bottom width is 5.5 m, and its depth is 2.8 m. - Drainage channel
The drainage channel's cross-section is an isosceles trapezoid whose bases are 1.80 m and 0.90 m long, and the arm is 0.60 meters long. Calculate the channel's depth. - Cross-section of a roof
The owner must cover the carport with a hipped roof with a rectangular cross-section of 8 m x 5 m. All roof surfaces have the same slope of 30°. Determine the price and weight of the roof if 1 m² cost €270 and weighs 43 kg.
- Z-score
The mean adult male pulse rate is 67.3 beats per minute, with a standard deviation of 10.3. Find the z-score for an adult male's pulse rate of 75. (Round the z-score to two decimal places. ) - Salami
We have six kinds of salami, six of which have ten pieces, and one of which has four pieces. How many ways can we distinctly choose five pieces of salami? - Cross-section 5721
Calculate how much electric current flows through the circuit if a 3 C (C is SI unit - Coulombs) electric charge passes through the conductor cross-section in 30 seconds.
