Pythagorean theorem - math word problems - page 51 of 73
Number of problems found: 1446
- Pyramidal 44061
A pyramidal candle with a square base has a side edge of s = 12 cm and a base edge of 4 cm. How much wax will we need to make it, and how long is the wick if it is 5% bigger than its height? - Regular quadrilateral pyramid
Find the surface area of a regular quadrilateral pyramid with a volume of 24 dm³ and a height of 45 cm. - Rotating cone
If the side of the rotating cone is 150 mm long and the circumference of the base is 43.96 cm, find its surface and volume. - Confectionery 7318
The confectioner needs to carve a cone-shaped decoration from a ball-shaped confectionery mass with a radius of 25 cm. Find the radius of the base of the ornament a (and the height h). He uses as much material as possible is used to make the ornament. - Rotating 28501
Which bags shaped like the shell of a rotating cone can hold the most popcorn? The first bag has a height of 20 cm, and the length of its side is 24 cm. The second bag has a base radius of 10 cm and a height of 25 cm. - Equilateral cone
A cup has the shape of an equilateral cone (side “s” is the same size as the diameter of its base - the axial section is an equilateral triangle) It is supposed to hold 0.2 liters of liquid at a level 1 cm below the rim. Calculate its diameter - Four-sided turret
The turret has the shape of a regular four-sided pyramid with a base edge 0.8 m long. The height of the turret is 1.2 m. How many square meters are needed to cover it, counting the extra 10% sheet metal waste? - Axial section of the cone
The axial section of the cone is an isosceles triangle in which the ratio of cone diameter to cone side is 2:3. Calculate its volume if you know its area is 314 cm square. - Faces diagonals
Find the cuboid volume if the cuboid's diagonals are x, y, and z (wall diagonals or three faces). Solve for x=1.6, y=1.8, z=1.6 - Pyramid-shaped roof
A block-shaped shed is covered with a quadrilateral pyramid-shaped roof with a base with sides of 6m and 3m and a height of 2.5m. How many m² (square meters) must be purchased if an extra 40% is calculated for roofing and waste? - Pyramid-shaped 7820
The pyramid-shaped tent has a square base with a side size of 2.2m and a height of 1.8m. How many square meters of tent canvas are needed to make it if we count an extra five percent for the foundation? - Calculate 5789
Calculate the volume and surface of the rotating cone with the base radius r = 4.6dm and the height v = 230mm. - Axial cut
The cone surface is 388.84 cm2, and the axial cut is an equilateral triangle. Find the cone volume. - Dimensions 44081
In the form of a pyramid on the house with a square floor plan, the roof has dimensions of 12 x 12 m, with a height of 2 m at the highest point. How much roofing do I need to buy? Count on a 10% reserve. - Observation tower
The observation tower is covered with a roof in the shape of a regular quadrangular pyramid with a base edge of 8 m and a height of 6 m. 60% of the roofing needs to be replaced. How many m² do you need to buy? - Felix
Calculate how much land Felix Baumgartner saw after jumping from 36 km above the ground. The radius of the Earth is R = 6378 km. - Quadrilateral pyramid
The height of a regular quadrilateral pyramid is 6.5 cm, and the angle between the base and the side wall is 42°. Calculate the surface area and volume of the body—round calculations to 1 decimal place. - Truncated cone 6
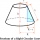Calculate the volume of the truncated cone whose bases consist of an inscribed circle and a circle circumscribed to the opposite sides of the cube with the edge length a=1. - Secret treasure
Scouts have a tent in the shape of a regular quadrilateral pyramid with a side of the base of 4 m and a height of 3 m. Find the container's radius r (and height h) so that they can hide the largest possible treasure. - Quadrilateral 29201 How much sheet is needed for a roof with the shape of a regular quadrilateral pyramid if its edge is 2.8 m long and the height of the roof is 0.8 m? Calculate 10% for the overlap (extra).
Do you have unsolved math question and you need help? Ask a question, and we will try to solve it. We solve math question.
