Geometry - math word problems - page 149 of 162
Number of problems found: 3232
- Percentage 80164
I was given a square ABCD 4.2 cm. Find the set of all points that have a distance less than or equal to 2 cm from one of its vertices and lie inside this square. Indicate how much of the square this area occupies as a percentage. - Spherical cap
From the sphere with a radius of 26 was a truncated spherical cap. Its height is 2. What part of the volume is a spherical cap from the whole sphere? - Average speed
What is the average speed you have to move around the world in 80 days? (Path along the equator, round to km/h). - CoG center
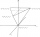Find the position of the center of gravity of a system of four mass points having masses, m1, m2 = 2 m1, m3 = 3 m1, and m4 = 4 m1, if they lie at the vertices of an isosceles tetrahedron. (in all case - Convex lens
The convex lens consists of two spherical segments (dimensions given in mm). Calculate its weight if the density of the glass is 2.5 g/cm³. Dimensions: 60mm in length and width of the upper part 5mm, the width of the lower part 8mm - Trapezoid MO-5-Z8
ABCD is a trapezoid in that lime segment CE is divided into a triangle and parallelogram. Point F is the midpoint of CE, the DF line passes through the center of the segment BE, and the area of the triangle CDE is 3 cm². Determine the area of the trapezoi - Cuboid and ratio
A cuboid has dimensions in a ratio of 1:2:6, and the surface area of the cuboid is 1000 dm². Calculate the volume of the cuboid. - Half-planes 36831
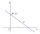The line p and the two inner points of one of the half-planes determined by the line p are given. Find point X on the line p so that the sum of its distances from points A and B is the smallest. - Equilateral triangle ABC
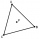In the equilateral triangle ABC, K is the center of the AB side, the L point lies on one-third of the BC side near point C, and point M lies on one-third of the side of the AC side closer to point A. Find what part of the ABC triangle contains the triangl - The resistance
What is the resistance of an aluminum wire, 0.2 km long and 10 mm in diameter? - Rectangle
In a rectangle with sides, 8 and 9 mark the diagonal. What is the probability that a randomly selected point within the rectangle is closer to the diagonal than any side of the rectangle? - Icerink
A rectangular rink with 68.7 m and 561 dm dimensions must be covered with a layer of ice 4.2 cm thick. How many liters of water is necessary for ice formation when the ice volume is 9.7% greater than the volume of water? - Center-symmetric 58201
Find out which we can write letters (uppercase) as center-symmetric. - Z8–I–5 MO 2019
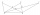For eight different points as shown in the figure, points C, D, and E lie on a line parallel to line AB, F is the midpoint of line AD, G is the midpoint of line AC, and H is the intersection of lines AC and BE. The area of triangle BCG is 12 cm² and the - Triangle-shaped 30011
Determine the map's scale if the 1.6 km, 2.4 km, and 2.7 km triangle-shaped forests are drawn on the map as a triangle with sides of 32 mm, 48 mm, and 54 mm. - Unit resistance
What is the resistance of a two-conductor line 10 m long made of 4.0 mm² aluminum wire? - Cylinder 72094
The tank has the shape of a rotating cylinder with a base radius of 6 m reach. Water flows at a speed of 2 liters per second for 3 hours. To what height does the water in the tank? - The tent
The tent has the shape of a regular square pyramid. The edge of the base is 3 m long, and the tent's height is 2 m. Calculate how much cover (without a floor) is used to make a tent. - Ice and water
We want to cover a rectangular rink with dimensions of 55 m and 25 m with a 4cm thick layer of ice. How many liters of water do we need if freezing water increases its volume by 10%? - Ladder
A 4 m long ladder touches the cube 1mx1m at the wall. How high reach on the wall?
Do you have unsolved math question and you need help? Ask a question, and we will try to solve it. We solve math question.
