Planimetrics - math word problems - page 155 of 184
Number of problems found: 3673
- Plan scale
The actual width of the room is 66 dm, and the width in the plan is 4.4 cm. What scale corresponds to the plan? - Map scale
The garden's plan shows a scale of 1:150, a width of 22 cm, and a length of 35 cm. What is its real area? - Diagonals
Construct a rhombus whose diagonals have lengths of 10cm and 8cm and make an angle of 115°. - Triangle and axes
Draw any triangle. Make the axis of its two sides. Their intersection is point S. (a) Measure the distance of point S from all three vertices (b) Draw the axis of the third side. - Rhombus EFGH
Construct the rhombus EFGH where e = 6.7cm, height to side h: vh = 5cm - Map scale
The rectangular plot has a scale of 1:10000 and an area of 3 cm² on the map. What area does this plot have on a 1:5000 scale map? - MO-Z5-3-66 tiles
The picture shows square tiles with a side of 10 dm, composed of four identical small rectangles and squares. The circumference of a small square is five times smaller than the circumference of the entire tile. Determine the dimensions of the rectangle. - Coordinates 32183
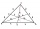The triangle ABC is given in the plane. A (-3,5), B (2,3), C (-1, -2) write the coordinates of the vectors u, v, w if u = AB, v = AC, and w = BC. Enter the coordinates of the centers of the lines SAB (..), SAC (...), SBC (. ..) - Construct 1
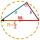Construct a triangle ABC, a = 7 cm, b = 9 cm with a right angle at C, and construct the axis of all three sides. Measure the length of side c (and write). - Construct 83195
Two line segments of different lengths are given. Construct a circle k so that both line segments are its chords. - Symmetry 13501
Draw a square KLMN, a point R that is a point of the square, and a point S that is not a point of this square. Draw the image of the square KLMN in central symmetry with the center : a) at point s b) at point M c) at point R - Triangle ABC
Construct a triangle ABC is given c = 60mm, hc = 40 mm and b = 48 mm analysis procedure steps construction - Midpoint of segment
Find the distance and midpoint between A(1,2) and B(5,5). - The intersection of the diagonals
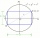In the rectangular coordinate system, a rectangle ABCD is drawn. These coordinates determine the vertices of the rectangle: A = (2.2) B = (8.2) C = (8.6) D = (2.6) Find the coordinates of the intersection of the diagonals of the ABCD rectangle. - Rectangular 24321 The rectangular garden on the 1:1000 scale plan is 10 cm by 15 cm. What is the perimeter of the garden?
- Course 21753
The course is 80m long. What will be its length on the plan at a scale of 1:500? (in cm) - Interested 18803
On the map, at a scale of 1:400 m and an area of 100 cm². How much are you interested in this land? - Floor
The room's floor area is 33 m2, and its width is 4 m. How many centimeters of circumference was measured on the floor on the map at the scale 1:75? - Z6–I–5 MO 2019
The shape in the picture was created by cutting a small cross out of a large cross. Each of these crosses can be composed of five identical squares, with the sides of the small squares being half the sides of the large squares. The area of the gray shap - Construct diagonals
The point B is a vertex of rectangle ABCD. The diagonal BD of this rectangle lies on the line p. Point X is an interior point of side AD of rectangle ABCD, and point Y is an internal point of side CD. Construct the missing vertices D, A, and C of the rect
Do you have unsolved math question and you need help? Ask a question, and we will try to solve it. We solve math question.
