Solid geometry, stereometry - page 105 of 121
Number of problems found: 2404
- Rectangle 7768
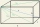The base of a cuboid is a rectangle. The ratio of its length to width is 3:2. The length of the rectangle of the base is in the ratio of 4:5 to the height of the block. The sum of the lengths of all the edges of the block is 2.8m. Find: a) the surface of - Axial section
The axial section of the cylinder is diagonal 45 cm long, and we know that the area of the side and the base area are in ratio 6:5. Calculate the height and radius of the cylinder base. - Hip-roof
The roof consists of two isosceles trapezoids and two isosceles triangles. The roof plan is a rectangle with dimensions of 8m and 14m, and the roof ridge is 8m long. The height of the trapezoid is 5m, the height of the triangles is 4.2m. How many tiles ar - Cone roof
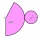How many m² of roofing is needed to cover a cone-shaped roof with a diameter of 10 m and a height of 4 m? Add an extra 4% to the overlays. - Seat
How much m² of fabric do we need to sew a 50cm-shaped cube-shaped seat if we add 10% of the material to the folds? - The roof
The roof has a spherical canopy with a base diameter of 8 m and a height of 2 m. Calculate the foil area with which the roof is covered when calculating 13% for waste and residues. - Liters od milk
The cylinder-shaped container contains 80 liters of milk. The milk level is 45 cm. How much milk will be in the container if the level rises to a height of 72 cm? - Perimeter 83259
The perimeter of the four-sided needle is 48 m, and its height is 2.5 m; how much will the sheet metal for this pyramid cost? If 1m² costs €1.5, a 12% loss due to joints and folds is included in the area. - How many
How many m² of copper sheet is needed to replace the roof of a conical tower with a diameter of 13 meters and a height of 24 meters if we count 8% of the material for bending and waste? - The observatory
The dome of the hemisphere-shaped observatory is 5.4 meters high. How many square meters of sheet metal need to be covered to cover it, and must we add 15 percent to the minimum amount due to joints and waste? - The body
The body has 2 m, 2 dm, and 10 cm dimensions. It weighs 28 kg. What is its density? - Perpendicular 68194
The closed box has the shape of a perpendicular prism with the base of an equilateral triangle. The edge of the base is 24 cm long, and the height of the box is 0.5 m. Calculate how many square meters of cardboard are needed to make 20 such boxes, assumin - Cylindrical 6636
A cylindrical container with a bottom diameter of 30 cm and a height of 20 cm is filled with water. We want to pour the water into another cylindrical container with a bottom diameter of 15 cm. What minimum height must the second container have for the wa - Painting
To paint the pool with dimensions: 2 meters depth, 3m x 4m we bought paint to 50 meters square. How much "paint" will be wasted? - Six-sided parasol
The parasol has the shape of the shell of a regular six-sided pyramid, whose base edge is a=6dm and height v=25cm. How much fabric is needed to make a parasol if we count 10% for joints and waste? - Leveling
Calculate how many we must purchase 25 kg bags of leveling concrete if we level room 19 m² to the "height" 11 mm if consumption is 1.7 kg per square meter and millimeter thickness. - Prism
The prism's base is a rhombus with a side 17 cm and a height 5 cm long. The height of the prism is 88% longer than the side length of the rhombus. Calculate the volume of the prism. - Conical bottle
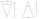When a conical bottle rests on its flat base, the water in the bottle is 8 cm from its vertex. When the same conical bottle is turned upside down, the water level is 2 cm from its base. What is the height of the bottle? - Four-sided turret
The turret has the shape of a regular four-sided pyramid with a base edge 0.8 m long. The height of the turret is 1.2 m. How many square meters are needed to cover it, counting the extra 10% sheet metal waste? - Pyramid-shaped roof
A block-shaped shed is covered with a quadrilateral pyramid-shaped roof with a base with sides of 6m and 3m and a height of 2.5m. How many m² (square meters) must be purchased if an extra 40% is calculated for roofing and waste?
Do you have unsolved math question and you need help? Ask a question, and we will try to solve it. We solve math question.
