Bearing - navigation
A ship travels 84 km on a bearing of 17° and then travels on a bearing of 107° for 135 km. Find the distance of the end of the trip from the starting point to the nearest kilometer.
Correct answer:
Showing 2 comments:
Dr Math
Q: A ship sailing on a bearing of 090⁰, its direction is?
A: A bearing of 90° is direction to the east.
north representing 0° or 360°
east representing 90°
south representing 180°
west representing 270°
A: A bearing of 90° is direction to the east.
north representing 0° or 360°
east representing 90°
south representing 180°
west representing 270°
3 years ago 1 Like
Tips for related online calculators
Our vector sum calculator can add two vectors given by their magnitudes and by included angle.
The Pythagorean theorem is the base for the right triangle calculator.
Do you want to round the number?
See also our trigonometric triangle calculator.
Try conversion angle units angle degrees, minutes, seconds, radians, grads.
The Pythagorean theorem is the base for the right triangle calculator.
Do you want to round the number?
See also our trigonometric triangle calculator.
Try conversion angle units angle degrees, minutes, seconds, radians, grads.
You need to know the following knowledge to solve this word math problem:
geometryarithmeticplanimetricsgoniometry and trigonometryUnits of physical quantitiesGrade of the word problem
Related math problems and questions:
- Plane II
A plane flew 50 km on a bearing of 63°20' and then flew in the direction of 153°20' for 140km. Find the distance between the starting point and the ending point. - Airport's 80482
The plane flew from airport m on a course of 132° to airport n, then from n to p on a course of 235°. The distance between the airport's mn is 380 km, np 284 km. What will be the return course to m, and what is the distance between the airport's pm? - In the desert
A man wondering in the desert walks 5.7 miles in the direction S 26° W. He then turns 90° and walks 9 miles in the direction N 49° W. At that time, how far is he from his starting point, and what is his bearing from his starting point? - A boy 5
A boy starts at A and walks 3km east to B. He then walks 4km north to C. Find the bearing of C from A.
- Aircraft bearing
Two aircraft will depart from the airport simultaneously. The first flight flies with a course of 30°, and the second with a course of 86°. Both fly at 330 km/h. How far apart will they be in 45 minutes of flight? - A radio antenna
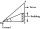Avanti is trying to find the height of a radio antenna on the roof of a local building. She stands at a horizontal distance of 21 meters from the building. The angle of elevation from her eyes to the roof (point A) is 42°, and the angle of elevation from - Expressway 5837
The expressway covers the distance from the starting point to the final station in 4 hours and 20 minutes. A passenger train, whose average speed is 30 km/h less, will cover this distance in 7 h 40 min. What is the speed of the express train, and what is
