Goniometry and trigonometry - math word problems - page 28 of 32
Number of problems found: 628
- Calculate pyramid
Calculate the volume of the pyramid, whose base edge a = 8 cm and the sidewall makes an angle α = 60° with the square base. - Prism diagonal
The body diagonal of a regular square prism has an angle of 60 degrees with the base, and the edge length is 10 cm. What is the volume of the prism? - SSA and geometry
The distance between the points P and Q was 356 m measured in the terrain. The viewer can see the PQ line at a 107°22' viewing angle. The observer's distance from P is 271 m. Find the viewing angle of P and the observer. - Calculate cone
The rotating cone has a base radius r = 226 mm, and the deviation of the side from the base plane is 56°. Calculate the height of the cone. - Power value
Describe how the instantaneous power value in the AC circuit changes during one period. - Determine the surface area
Find the surface area of a cone of height 30 cm whose side makes an angle of 60° with the base plane. - Pyramid 8
Calculate the volume and the surface area of a regular quadrangular pyramid with a base side of 9 cm and a side wall with the base has an angle of 75°. - Diagonals of a prism
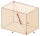The base of the square prism is a rectangle with dimensions of 3 dm and 4 dm. The height of the prism is 1 m. Find out the angle between the body diagonal and the base's diagonal. - Tetrahedral pyramid
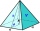Calculate the regular tetrahedral pyramid's volume and surface if the area of the base is 20 cm² and the deviation angle of the side edges from the plane of the base is 60 degrees. - Cuboid
Cuboid ABCDEFGH with 10 cm height has a base edge length 6 cm and 8 cm. Determine the angle between the body diagonal and the base plane (round to degrees). - Rotary cone
The volume of the rotation of the cone is 733 cm³. The angle between the side of the cone and the base angle is 75°. Calculate the lateral surface area of this cone. - Quadrilateral pyramid
The height of a regular quadrilateral pyramid is 6.5 cm, and the angle between the base and the side wall is 42°. Calculate the surface area and volume of the body—round calculations to 1 decimal place. - Block
Calculate the volume of a cuboid ABCDEFGH if |AB| = 7 cm, |BC| = 8 cm and the angle ∠CDG = 30.1° - Spherical section cut
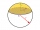Find the volume of a spherical section if the radius of its base is 10 cm and the magnitude of the central angle ω = 120 degrees. - Quadrilateral pyramid
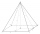The regular quadrilateral pyramid has a base edge a = 1.56 dm and a height h = 2.05 dm. Calculate: a) the deviation angle of the sidewall plane from the base plane b) deviation angle of the side edge from the plane of the base - Quadrilateral prism
Calculate the volume and surface area of a regular quadrilateral prism with base edge a=24 cm if the body diagonal makes an angle of 66° with the base. - 4side pyramid
Calculate the volume and surface of the regular four-sided pyramid whose base edge is 4 cm long. The angle from the plane of the sidewall and base plane is 60 degrees. - Climb
The road sign that informs the climb is 10.3%—the car drives 10 km along this road. What is the height difference that the car went? - Side deviation
Frustum has the base radii of the figures r1 and r2: r1> r2, r2 = s, and if the side deviation from the base plane is 60°. Express the surface and volume of the cone frustum using its side s. - Octagonal pyramid
Find the volume of a regular octagonal pyramid with height v = 100 and the angle of the side edge with the base plane is α = 60°.
Do you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
