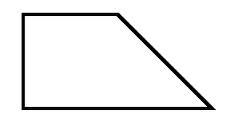
Trapezoid MO
The rectangular trapezoid ABCD with the right angle at point B, |AC| = 12, |CD| = 8, diagonals are perpendicular to each other.
Calculate the perimeter and area of the trapezoid.
Calculate the perimeter and area of the trapezoid.
Correct answer:
Tips to related online calculators
Looking for help with calculating roots of a quadratic equation?
Need help to calculate sum, simplify or multiply fractions? Try our fraction calculator.
Do you have a linear equation or system of equations and looking for its solution? Or do you have a quadratic equation?
Pythagorean theorem is the base for the right triangle calculator.
See also our trigonometric triangle calculator.
Need help to calculate sum, simplify or multiply fractions? Try our fraction calculator.
Do you have a linear equation or system of equations and looking for its solution? Or do you have a quadratic equation?
Pythagorean theorem is the base for the right triangle calculator.
See also our trigonometric triangle calculator.
You need to know the following knowledge to solve this word math problem:
Related math problems and questions:
- Ratio iso triangle
The ratio of the sides of an isosceles triangle is 7:6:7. Find the base angle to the nearest answer correct to 3 significant figures. - Right triangle eq2
Find the lengths of the sides and the angles in the right triangle. Given area S = 210 and perimeter o = 70. - Rectangular trapezoid
Calculate the area of a rectangular trapezoid with a right angle at point A and if |AC| = 4 cm, |BC| = 3 cm and the diagonal AC is perpendicular to the side BC. - Triangle in a square
In a square ABCD with side a = 6 cm, point E is the center of side AB, and point F is the center of side BC. Calculate the size of all angles of the triangle DEF and the lengths of its sides. - Right
Determine angles of the right triangle with the hypotenuse c and legs a, b, if: 3a +4b = 4.9c - Right triangle
Calculate the missing side b and interior angles, perimeter, and area of a right triangle if a=10 cm and hypotenuse c = 16 cm. - The aspect ratio
The aspect ratio of the rectangular triangle is 13: 12: 5. Calculate the internal angles of the triangle. - Rectangle 49153
Rectangle ABCD, whose | AB | = 5cm, | AC | = 8 cm, ∢ | CAB | = 30 °. How long is the other party and what is its area? - Trapezoid: 18703
In the ABCD trapezoid: | AD | = | CD | = | BC | a | AB | = | AC |. Determine the size of the delta angle. - Space diagonal angles
Calculate the angle between the body diagonal and the side edge c of the block with dimensions: a = 28cm, b = 45cm and c = 73cm. Then, find the angle between the body diagonal and the plane of the base ABCD. - Trapezoid IV
In a trapezoid ABCD (AB||CD) is |AB| = 15cm |CD| = 7 cm, |AC| = 12 cm, AC is perpendicular to BC. What area has a trapezoid ABCD? - Rhombus diagonals
In the rhombus ABCD are given the sizes of diagonals e = 24 cm; f = 10 cm. Calculate the side length of the diamond and the size of the angles, calculate the area of the diamond. - Angle of diagonals
Calculate the perimeter and the area of a rectangle if its diagonal is 14 cm and the diagonals form an angle of 130°. - Side wall planes
Find the volume and surface of a cuboid whose side c is 30 cm long and the body diagonal forms angles of 24°20' and 45°30' with the planes of the side walls. - Diagonal
The rectangular ABCD trapeze, whose AD arm is perpendicular to the AB and CD bases, has an area of 15 cm square. Bases have lengths AB = 6cm, CD = 4cm. Calculate the length of the AC diagonal. - Coordinates of square vertices
I have coordinates of square vertices A / -3; 1/and B/1; 4 /. Find coordinates of vertices C and D, C and D. Thanks, Peter. - Diagonals of pentagon
Calculate the diagonal length of the regular pentagon: a) inscribed in a circle of radius 12dm; b) a circumscribed circle with a radius of 12dm.
