Find the 5
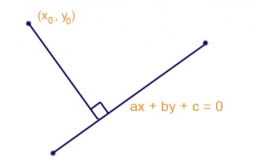
Find the equation of the circle with the center at (1,20), which touches the line 8x+5y-19=0
Correct answer:
Showing 1 comment:
Dr Math
Hint - use formula for Distance Between a Point and a Line = which is radius of circle
5 years ago 2 Likes
Tips for related online calculators
Our vector sum calculator can add two vectors given by their magnitudes and by included angle.
See also our right triangle calculator.
See also our trigonometric triangle calculator.
See also our right triangle calculator.
See also our trigonometric triangle calculator.
You need to know the following knowledge to solve this word math problem:
Related math problems and questions:
- Circle
The circle touches two parallel lines, p, and q, and its center lies on line a, which is the secant of lines p and q. Write the equation of the circle and determine the coordinates of the center and radius. p: x-10 = 0 q: -x-19 = 0 a: 9x-4y+5 = 0 - Circle
Write the equation of a circle that passes through the point [0,6] and touches the X-axis point [5,0]: (x-x_S)²+(y-y_S)²=r² - Applies 14683
Point B is the center of the circle. The line AC touches the circles at point C and applies AB = 20 cm and AC = 16 cm. What is the radius of the circle BC? - Sphere equation
Obtain the equation of a sphere. Its center is on the line 3x+2z=0=4x-5y and passes through the points (0,-2,-4) and (2,-1,1). - Circle
From the equation of a circle: -x² -y² +16x -4y -59 = 0 Calculate the coordinates of the center of the circle S[x0, y0] and the radius of the circle r. - Prove
Prove that k1 and k2 are the equations of two circles. Find the equation of the line that passes through the centers of these circles. k1: x²+y²+2x+4y+1=0 k2: x²+y²-8x+6y+9=0 - Tangents to ellipse
Find the magnitude of the angle at which the ellipse x² + 5 y² = 5 is visible from the point P[5, 1]. - Polynomial: 8465
Solve the equation with the polynomial: x / 2 + 1/2 + 3 = x - On line
On line p: x = 4 + t, y = 3 + 2t, t is R, find point C, which has the same distance from points A [1,2] and B [-1,0]. - What is 19
What is the equation of the line whose x-intercept is - 3 and y-intercept is -4? Find coefficients A, B, C in normal line equation: Ax + By = C - Center
Calculate the coordinates of the circle center: x² -4x + y² +10y +25 = 0 - Parametrically 6400
Find the angle of the line, which is determined parametrically x = 5 + t y = 1 + 3t z = -2t t belongs to R and the plane, which is determined by the general equation 2x-y + 3z-4 = 0. - Find parameters
Find parameters of the circle in the plane - coordinates of center and radius: x²+(y-3)²=14 - Perpendicular
Find the slope of the line perpendicular to the line p: y = 8x +6. - Equation of the circle
Find the equation of the circle inscribed in the rhombus ABCD where A[1, -2], B[8, -3], and C[9, 4]. - Find the 10
Find the value of t if 2tx+5y-6=0 and 5x-4y+8=0 are perpendicular and parallel. What angle does each line make with the x-axis, and find the angle between the lines? - Intersection of Q2 with line
The equation of a curve C is y=2x² - 8x +9, and the equation of a line L is x + y=3. (1) Find the x-coordinates of the points of intersection of L and C. (ii) show that one of these points is also the
