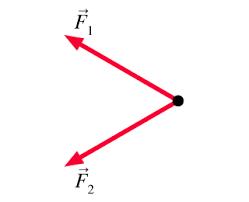
Two forces
The two forces, F1 = 580N and F2 = 630N, have an angle of 59 degrees. Calculate their resultant force, F.
Correct answer:
Showing 1 comment:
Dr Math
checkout our new two vector sum calculator:
https://www.hackmath.net/en/calculator/sum-of-the-two-vectors?a=580&b=630&a1=59&submit=1
https://www.hackmath.net/en/calculator/sum-of-the-two-vectors?a=580&b=630&a1=59&submit=1
Tips for related online calculators
Our vector sum calculator can add two vectors given by their magnitudes and by included angle.
See also our right triangle calculator.
See also our trigonometric triangle calculator.
See also our right triangle calculator.
See also our trigonometric triangle calculator.
You need to know the following knowledge to solve this word math problem:
- geometry
- analytic geometry
- vector
- algebra
- expression of a variable from the formula
- planimetrics
- Pythagorean theorem
- right triangle
- circle
- triangle
- rhomboid
- goniometry and trigonometry
- sine
- cosine
Units of physical quantities:
Grade of the word problem:
Related math problems and questions:
- Resultant force
Calculate mathematically and graphically the resultant of three forces with a common center if: F1 = 50 kN α1 = 30° F2 = 40 kN α2 = 45° F3 = 40 kN α3 = 25° - Mass point
Two equal forces of 30 Newtons act on a mass point. Find the magnitude of the resultant force if these forces form an angle of 42°. - Forces
Determine the resultant of two perpendicular forces F1 = 560 N and the second force 25% smaller. - Two forces 3
Two forces with magnitudes 8 Newtons and 15 Newtons act at a point. Find the angle between the forces if the resultant force is 17 Newtons.
- Forces
Forces with magnitudes F1 = 42N and F2 = 35N act at a common point and make an angle of 77°12'. How big is their resultant? - Two forces
Two forces with magnitudes of 25 and 30 pounds act on an object at 10° and 100° angles. Find the direction and magnitude of the resultant force. Round to two decimal places in all intermediate steps and your final answer. - Perpendicular 81758
Distribute the force of magnitude F = 100 N into two perpendicular components with magnitudes F1 and F2 so that the angle between forces F1 and F is 43°52'.
