Geometry - math word problems - page 15 of 158
Number of problems found: 3148
- Calculate 80741
In the camp, the children made a mock-up of the campsite. In its center was a maple, which had a height of 28 cm on the model. Calculate the height of the maple in the campsite if, in the morning, it casts a shadow 14 m long and its model has a shadow 49 - College 2
The college student is moving into a dormitory. The student rents a truck for $19.95 plus $0.99 per mile. Before returning the truck, the student fills the tank with gasoline, which costs $65.32. The total cost was $144.67. Using a linear equation, explai - Inner angles
The magnitude of the internal angle at the central vertex C of the isosceles triangle ABC is 72°. The line p, parallel to the base of this triangle, divides the triangle into a trapezoid and a smaller triangle. How big are the inner angles of the trapezoi - Sleep vs. watch TV time
Using a data set relating to the number of episodes I watch on TV in a day (x) versus the number of hours of sleep I get that night (y), I construct the linear model y=−0.6x+11 Which of the following general observations can you make from this model? a) I
- Tachometer
Tatra's tachometer shows the initial state 886123 km this morning. Today, Tatra travels at an average speed of 44 km/h. Determine the function that describes the Tatra's tachometer depending on the time. What is the state of the tachometer after 4 hours? - Coordinates
Determine the coordinates of the vertices and the area of the parallelogram, the two sides of which lie on the lines 8x + 3y + 1 = 0, 2x + y-1 = 0, and the diagonal on the line 3x + 2y + 3 = 0 - Ruler
Peter is looking at John over a ruler that keeps at an arm's distance of 60 cm from the eye, and on the ruler, John measured the height of 15 mm. John is 2 meters high. How far from Peter stands John? - Circle
The circle touches two parallel lines, p, and q, and its center lies on line a, which is the secant of lines p and q. Write the equation of the circle and determine the coordinates of the center and radius. p: x-10 = 0 q: -x-19 = 0 a: 9x-4y+5 = 0 - Hexagon in circle
Calculate the radius of a circle whose length is 10 cm greater than the circumference of a regular hexagon inscribed in this circle.
- Calculate 82282
Calculate the sizes of the interior angles in the triangle whose vertices are the points marked by the numbers 1, 5, and 8 on the clock face. - Trapezoid 20873
In the trapezoid ABCD (AB II CD) is α = 57 °, γ = 4β. Calculate the size of all interior angles. - Similar triangles
Triangle A'B'C 'is similar to triangle ABC, whose sides are 5 cm, 8 cm, and 7 cm long. What is the length of the sides of the triangle A'B'C' if its circumference is 80 cm? - On line
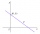On line p: x = 4 + t, y = 3 + 2t, t is R, find point C, which has the same distance from points A [1,2] and B [-1,0]. - Reverse Pythagorean theorem
Given are the lengths of the sides of the triangles. Decide which one is rectangular: Δ ABC: 66 dm, 60 dm, 23 dm ... Δ DEF: 20 mm, 15 mm, 25 mm ... Δ GHI: 16 cm, 20 cm, 12 cm ... Δ JKL: 58 cm, 63 cm, 23 cm ... Δ MNO: 115 mm,
- Calculate 16223
The following elements are known in the right triangle ABC: a = 10 cm, height to side c h = 9.23 cm. Calculate o, R (radius of the inscribed circle), r (radius of the inscribed circle). - Two similar
There are two similar triangles. One has a circumference of 100 cm, and the second has sides successively 8 cm, 14 cm, and 18 cm longer than the first. Find the lengths of its sides. - Sides of right angled triangle
One leg is 1 m shorter than the hypotenuse, and the second leg is 2 m shorter than the hypotenuse. Find the lengths of all sides of the right-angled triangle. - Tangent
What distance are the tangent t of the circle (S, 4 cm) and the chord of this circle, which is 6 cm long and parallel to the tangent t? - Right angled triangle 2
LMN is a right-angled triangle with vertices at L(1,3), M(3,5), and N(6,n). Given angle LMN is 90° find n
Neziswa is conducting an experiment in which the temperature is measured carefully. The temperature was 76°C at the end of the first minute, and then it fell by 5°C every minute. Determine a formula to calculate the temperature (T) after m minutes.Do you have unsolved problem that you need help? Ask a question, and we will try to solve it. Solving math problems.
