Goniometry and trigonometry - math word problems - page 12 of 32
Number of problems found: 624
- Parallelogram diagonals
Find the area of a parallelogram if the diagonals u1 = 15 cm and u2 = 12 cm and the angle formed by them is 30 degrees. - Viewing angle
The observer sees a straight fence 60 m long at a viewing angle of 30°. It is 102 m away from one end of the enclosure. How far is the observer from the other end of the enclosure? - Observation tower
The observation tower has a height of 105 m above sea level. The ship is aimed at a depth angle of 1° 49' from the tower. How far is the ship from the base of the tower? - Power line pole
From point A, the power pole is visible at an angle of 18 degrees. From place B, which we reach if we go from place A 30m towards the pillar at an angle of 10 degrees. Find the height of the power pole. - Raindrops
The train runs at a speed of 14 m/s, and raindrops draw lines on the windows, forming an angle of 60 degrees with the horizontal. What speed do drops fall? - Diagonals of pentagon
Calculate the diagonal length of the regular pentagon: a) inscribed in a circle of radius 12dm; b) a circumscribed circle with a radius of 12dm. - Loonie
Loonie has three wooden sticks measuring 17 inches, 21 inches, and 25 inches. He lays them down to form a triangle. Find the measure of the angle enclosed by 17 inches and 21 inches. (Express answers to the nearest hundredths) (using the law of cosines) - Magnitude 25411
There is a circle with a radius of 10 cm and its chord, which is 12 cm long. Calculate the magnitude of the central angle that belongs to this chord. - The pond
We can see the pond at an angle of 65°37'. Its endpoints are 155 m and 177 m away from the observer. What is the width of the pond? - Triangle's centroid
In the triangle ABC the given lengths of its medians tc = 9, ta = 6. Let T be the intersection of the medians (triangle's centroid), and the point S is the center of the side BC. The magnitude of the CTS angle is 60°. Calculate the length of the BC side t - Clouds
From two points, A and B, on the horizontal plane, a forehead cloud was observed above the two points under elevation angles 73°20' and 64°40'. Points A and B are separated by 2830 m. How high is the cloud? - Isosceles 83247
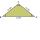Calculate the lengths of the sides in an isosceles triangle, given the height (to the base) Vc= 8.8cm and the angle at the base alpha= 38°40`. - The bases
The bases of the isosceles trapezoid ABCD have 10 cm and 6 cm lengths. Its arms form an angle α = 50˚ with a longer base. Calculate the circumference and area of the ABCD trapezoid. - Shooter
The shooter fired at a target from a distance 49 m. The individual concentric circle of targets has radius increments of 1 cm (25 points) by 1 point. The shot was shifted by 16' (angle degree minutes). How many points should he win his shot? - In the desert
A man wondering in the desert walks 5.7 miles in the direction S 26° W. He then turns 90° and walks 9 miles in the direction N 49° W. At that time, how far is he from his starting point, and what is his bearing from his starting point? - Determine 8202
An observer watches two boats at depth angles of 64° and 48° from the top of the hill, which is 75 m above the lake level. Determine the distance between the boats if both boats and the observer are in the same vertical plane. - Cosine
Cosine and sine theorem: Calculate all unknown values (side lengths or angles) from triangle ABC. c = 2.9 cm; β = 28°; γ = 14° α =? °; a =? cm; b =? cm - Diagonal BD
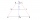Find the length of the diagonal BD in a rectangular trapezoid ABCD with a right angle at vertex A when/AD / = 8,1 cm and the angle DBA is 42° - Parallelogram
Find the parallelogram's perimeter, where base a = 8 cm, height v = 3 cm, and angle alpha = 35° is the magnitude of the angle at vertex A. - TV tower
Calculate the height of the television tower if an observer standing 430 m from the base of the tower sees the peak at an altitude angle of 23°.
Do you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
