Planimetrics - math word problems - page 133 of 184
Number of problems found: 3667
- Two cyclists
 Two cyclists started crossing at the same time. One goes to the north speed of 20 km/h, the second eastward at a speed of 26 km/h. What will be the direct distance cycling 30 minutes from the start?
Two cyclists started crossing at the same time. One goes to the north speed of 20 km/h, the second eastward at a speed of 26 km/h. What will be the direct distance cycling 30 minutes from the start? - Wipes
 The mummy wiped out the square wipes, and the veil was next to each other on the cord stretched out between the two trees. She used a cord of 7.5 meters in length, requiring about 8 dm on each side of the trunk. All wipes are 45 cm wide. The mummy leaves
The mummy wiped out the square wipes, and the veil was next to each other on the cord stretched out between the two trees. She used a cord of 7.5 meters in length, requiring about 8 dm on each side of the trunk. All wipes are 45 cm wide. The mummy leaves - Ratio of squares
 A circle is given, and a square is inscribed. The smaller square is inscribed in a circular arc formed by the square's side and the circle's arc. What is the ratio of the areas of the large and small squares?
A circle is given, and a square is inscribed. The smaller square is inscribed in a circular arc formed by the square's side and the circle's arc. What is the ratio of the areas of the large and small squares? - Calculate 70814
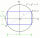 The lengths of the sides AB and AD of the rectangle ABCD are in the ratio 3:4. A circle k with a diameter of 10 cm describes a rectangle. Calculate the side lengths of a given rectangle.
The lengths of the sides AB and AD of the rectangle ABCD are in the ratio 3:4. A circle k with a diameter of 10 cm describes a rectangle. Calculate the side lengths of a given rectangle. - Percentage - rhombus
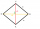 If the rhombus side in the image increases by half, by what percentage will the area of the rhombus increase?
If the rhombus side in the image increases by half, by what percentage will the area of the rhombus increase? - It is rectangular?
 The size of two of the angles in a triangle is α=110°, β=40°. Is it a right triangle?
The size of two of the angles in a triangle is α=110°, β=40°. Is it a right triangle? - Perimeter 27471
 The Ferris wheel in London has a diameter of 135 meters, and one turn takes about 30 minutes. At what speed per second do the cabins move around the perimeter of the London London Eye?
The Ferris wheel in London has a diameter of 135 meters, and one turn takes about 30 minutes. At what speed per second do the cabins move around the perimeter of the London London Eye? - Largest squares
 How many of the largest square sheets did the plumber cut the honeycomb from 16 dm and 96 dm?
How many of the largest square sheets did the plumber cut the honeycomb from 16 dm and 96 dm? - The parabolic segment
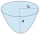 The parabolic segment has a base a = 4 cm and a height v = 6 cm. Calculate the volume of the body that results from the rotation of this segment a) around its base b) around its axis.
The parabolic segment has a base a = 4 cm and a height v = 6 cm. Calculate the volume of the body that results from the rotation of this segment a) around its base b) around its axis. - A rectangle 2
 A rectangle has a diagonal length of 74cm. Its side lengths are in a ratio of 5:3. Find its side lengths.
A rectangle has a diagonal length of 74cm. Its side lengths are in a ratio of 5:3. Find its side lengths. - Isosceles trapezoid
 Calculate the area of an isosceles trapezoid whose bases are in the ratio of 4:3; leg b = 13 cm and height = 12 cm.
Calculate the area of an isosceles trapezoid whose bases are in the ratio of 4:3; leg b = 13 cm and height = 12 cm. - Calculate 73024
 Calculate the permille descent of the railway line in the section of 7.2 km by 21.6 m.
Calculate the permille descent of the railway line in the section of 7.2 km by 21.6 m. - Similarity
 The area of the regular 5-gon is 495 cm². The area of similar 5-gon is 606 dm². What is the coefficient of similarity?
The area of the regular 5-gon is 495 cm². The area of similar 5-gon is 606 dm². What is the coefficient of similarity? - Aircraft bearing
 Two aircraft will depart from the airport simultaneously. The first flight flies with a course of 30°, and the second with a course of 86°. Both fly at 330 km/h. How far apart will they be in 45 minutes of flight?
Two aircraft will depart from the airport simultaneously. The first flight flies with a course of 30°, and the second with a course of 86°. Both fly at 330 km/h. How far apart will they be in 45 minutes of flight? - Perpendicular 5712
 Adam and Boris go from school on two perpendicular paths. Adam's average speed is 6 km/h, and Borisova's is 8 km/h. How far will they be by air for 0.5 hours?
Adam and Boris go from school on two perpendicular paths. Adam's average speed is 6 km/h, and Borisova's is 8 km/h. How far will they be by air for 0.5 hours? - Constant Angular Acceleration
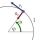 The particle began to move from rest along a circle with a constant angular acceleration. After five cycles (n = 5), its angular velocity reached the value ω = 12 rad/s. Calculate the magnitude of the angular acceleration ε of this motion and the time int
The particle began to move from rest along a circle with a constant angular acceleration. After five cycles (n = 5), its angular velocity reached the value ω = 12 rad/s. Calculate the magnitude of the angular acceleration ε of this motion and the time int - Right-angled 81019
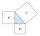 In the right-angled triangle ABC (AB is the hypotenuse), a : b = 24 : 7, and the height to the side c = 12.6 cm applies. Calculate the lengths of the sides of triangle ABC.
In the right-angled triangle ABC (AB is the hypotenuse), a : b = 24 : 7, and the height to the side c = 12.6 cm applies. Calculate the lengths of the sides of triangle ABC. - Clock hands
 The hands-on clock shows the time as 12 hours and 2 minutes. Three hours later, calculate the size of an acute angle between the clock hands.
The hands-on clock shows the time as 12 hours and 2 minutes. Three hours later, calculate the size of an acute angle between the clock hands. - Ray
 Light ray loses 1/19 of brightness passing through a glass plate. What is the brightness of the ray after passing through 5 identical plates?
Light ray loses 1/19 of brightness passing through a glass plate. What is the brightness of the ray after passing through 5 identical plates? - Diver
 Please calculate using Pascal's law. The window of the diving helmet has a surface area of about 7dm². What pressure force acts on the window at a depth of 20 meters below the water surface?
Please calculate using Pascal's law. The window of the diving helmet has a surface area of about 7dm². What pressure force acts on the window at a depth of 20 meters below the water surface?
Do you have unsolved math question and you need help? Ask a question, and we will try to solve it. We solve math question.
