The Law of Cosines - practice problems - page 3 of 5
Direction: Solve each problem carefully and show your solution in each item.Number of problems found: 83
- Cosine
Cosine and sine theorem: Calculate all unknown values (sides and angles) of the triangle ABC. a = 20 cm; b = 15 cm; γ = 90°; c =? cm; α =? °; β =? ° - Circumscribed circle ABC
Triangle ABC, with sides a = 15 cm, b = 17.4 cm, and c = 21.6 cm, is circumscribed by a circle. Calculate the area of the segments determined by the sides of the triangle. - Triangle's centroid
In the triangle ABC the given lengths of its medians tc = 9, ta = 6. Let T be the intersection of the medians (triangle's centroid), and the point S is the center of the side BC. The magnitude of the CTS angle is 60°. Calculate the length of the BC side t - The pond
We can see the pond at an angle of 65°37'. Its endpoints are 155 m and 177 m away from the observer. What is the width of the pond? - Loonie
Loonie has three wooden sticks measuring 17 inches, 21 inches, and 25 inches. He lays them down to form a triangle. Find the measure of the angle enclosed by 17 inches and 21 inches. (Express answers to the nearest hundredths) (using the law of cosines) - Viewing angle
The observer sees a straight fence 60 m long at a viewing angle of 30°. It is 102 m away from one end of the enclosure. How far is the observer from the other end of the enclosure? - Parallelogram 65334
In a parallelogram, the sum of the lengths of the sides a+b = 234. The angle subtended by the sides a and b is 60°. The diagonal size against the given angle of 60° is u=162. Calculate the sides of the parallelogram, its perimeter, and its area. - Determine 81756
The area for shooting training has the shape of a trapezoid, the parallel sides of which are 36m, 21m long, and the remaining sides are 14m, 16m long. Determine the size of the interior angles with a longer base. - Heron backlaw
Calculate the unknown side in a triangle with sides 39 and 38 and area 438.6. - Parallelogram
The sides of the parallelogram are 8 cm and 6 cm long, and the diagonals' angle is 60°. What is its area? - Diagonals in diamond
In the rhombus, a = 160 cm and alpha = 60 degrees are given. Calculate the length of the diagonals. - Magnitudes 64704
The triangle ABC determines the size of the sides a and b and the magnitudes of the interior angles β and γ, given c = 1.86 m, the line on the side c is 2.12 m, and the angle alpha is 40 ° 12 '. - Inner angles
The inner angles of the triangle are 30°, 45°, and 105° and its longest side is 10 cm. Calculate the shortest side length, and write the result in cm up to two decimal places. - Parallelogram - area
Calculate the area of the parallelogram if the sides are a = 80, b = 60 long, and the size of the diagonal angle is 60°. - Calculate 82696
In the triangle ABC, b=5 cm, c=6 cm, /BAC/ = 80° are given. Calculate the sizes of the other sides and angles, and further determine the sizes of the tangent tc and the area of the triangle. - Circular railway
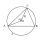The railway connects points A, B, and C in a circular arc, whose distances are | AB | = 30 km, AC = 95 km, and BC | = 70 km. How long will the track be from A to C? - Triangle SAS
Calculate the triangle area and perimeter if the two sides are 46 m and 33 m long and angle them clamped is 170 °. - Aircraft bearing
Two aircraft will depart from the airport simultaneously. The first flight flies with a course of 30°, and the second with a course of 86°. Both fly at 330 km/h. How far apart will they be in 45 minutes of flight? - The cosine law
Solve the unknown dimensions for the following triangle: Triangle ABC: Angle A=43 degrees, b=7.0cm, c=6.0cm Question 1. Angle B with units written as degrees Question 2. Angle C with units written as degrees Question 3. a, rounded to the nearest tenth of - Two groves
Two groves A and B are separated by a forest. Both are visible from the hunting grove C, which is connected to both by direct roads. What will be the length of the projected road from A to B if AC = 5004 m, BC = 2600 m, and angle ABC = 53° 45'?
Do you have unsolved math question and you need help? Ask a question, and we will try to solve it. We solve math question.
