Planimetrics - math word problems - page 141 of 184
Number of problems found: 3667
- Inscribed triangle
A circle is an inscribed triangle, and its vertices divide the circle into three arcs. The length of the arcs is in the ratio 2:3:7. Find the interior angles of a triangle. - Similarity coefficient
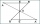In the triangle TMA, the length of the sides is t = 5cm, m = 3.5cm, and a = 6.2cm. Another similar triangle has side lengths of 6.65 cm, 11.78 cm, and 9.5 cm. Determine the similarity coefficient of these triangles and assign similar sides to each other. - Triangle
In triangle ABC, there is a point S with the center of the inscribed circle. The area of quadrilateral ABCS is equal to four-fifths of the area of triangle ABC. The lengths of the sides of triangle ABC expressed in centimeters are all integers and the - Circumferential angle
Vertices of the triangle ΔABC lay on the circle and are divided into arcs in the ratio 10:8:7. Determine the size of the angles of the triangle ΔABC. - Rhombus construction
Construct ABCD rhombus if its diagonal AC=9 cm and side AB = 6 cm. Inscribe a circle in it, touching all sides. - Sides ratio
Calculate the circumference of a triangle with an area of 84 cm² if a:b:c = 10:17:21 - Actually 83215
The square-shaped plot actually covers an area of 81 ares. Maybe draw it in the village plan on a scale of 1:200. What will be the length of the side on the plan? - Snowman's 82155
Under the column, the children built a 1.65m tall snowman. The snowman's shadow is 135 cm long. The shadow of the column has a length of 4.05 m. How tall is the pole? - Motorcycle 5354
The diameter of the motorcycle wheel is 60 cm. How many times does the wheel turn on a track 1,884 km long? - Rectangle vs square
One side of the rectangle is 1 cm shorter than the side of the square. The second side is 3 cm longer than the side of the square. The square and rectangle have the same area. Calculate the length of the sides of a square and a rectangle. - Two conductors
Two conductors have resistance Rs=5 ohms when connected in series and Rp=1.2 ohms when connected in parallel. Calculate the value of the resistors. - Similarity coefficient
Given triangle ABC with sides a = 12 cm b = 9 cm c = 7 cm and triangle DEF with sides d = 8.4 cm, e = 6.3 cm f = 4.9 cm Find out if triangles ABC and DEF are similar if so, write the similarity coefficient and according to which sentence they are similar - Lookout tower
Calculate the height of a lookout tower forming a shadow of 36 m if a column 2.5 m high has a shadow of 1.5 m simultaneously. - Chimney and tree
Calculate the height of the factory chimney, which casts a shadow of 6.5 m long in the afternoon. At the same time, a 6 m high tree standing near it casts a shadow 25 dm long. - An electrician 3
In setting up a wiring job, an electrician ran the following lengths of wire: 340 m, 203 m, 74 m, and 99 m. Find the total length of wire used. - Clock Tower
What angle is between the hands-on Clock Tower when it shows 17 hours and 35 minutes? - Bases
The length of the bases trapezium is in ratio 2:4. The length of the midline is 20. How long are the bases of a trapezoid? - Distances 79974
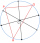The picture shows three villages, A, B, and C, and their mutual air distances. The new straight railway line is to be built so that all the villages are the same distance from the line and that this distance is the smallest possible. How far will they be - The chimney
The chimney casts a shadow 45 meters long. The one-meter-long rod standing perpendicular to the ground has a shadow 90 cm long. Calculate the height of the chimney. - Trapezium diagonals
It is given trapezium ABCD with bases | AB | = 12 cm, |CD| = 8 cm. Point S is the intersection of the diagonals for which |AS| is 6 cm long. Calculate the length of the full diagonal AC.
Do you have unsolved math question and you need help? Ask a question, and we will try to solve it. We solve math question.
