Planimetrics - math word problems - page 170 of 184
Number of problems found: 3667
- View angle - river
The 15 m high building is 30 m away from the river bank. The river's width can be seen from the roof of this building at an angle of 15°. How wide is the river? - Arc and segment
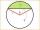Calculate the length of circular arc l, the area of the circular arc S1, and the area of circular segment S2. The circle's radius is 88, and the corresponding angle is (4)/(7) π. - Sides of the triangle
The sides of the triangle ABC have a length of 4 cm, 5 cm, and 7 cm. Construct triangle A'B'C', similar to triangle ABC, which has a circumference of 12 cm. - Calculate 60993
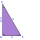In the right triangle ABC, calculate the magnitude of the interior angles if / AB / = 13 cm; / BC / = 12 cm and / AC / = 5 cm. - Big tree
You are standing 20 feet away from a tree, and you measure the elevation angle to be 38°. How tall is the tree? - Triangle 75
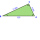Triangle ABC has angle C bisected and intersected AB at D. Angle A measures 20 degrees, and angle B measures 40 degrees. The question is to determine AB-AC if length AD=1. - An isosceles trapezoid
An isosceles trapezoid has base angles of 50° each, and its bases are 20 cm and 30 cm. Compute its area. - Isosceles triangle
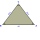Calculate the size of the interior angles and the length of the base of the isosceles triangle if the arm's length is 17 cm and the height of the base is 12 cm. - The ladder
The ladder has a length of 3 m and is leaning against the wall, and its inclination to the wall is 45°. How high does it reach? - Parallelogram - area
Calculate the area of the parallelogram if a = 57cm, the diagonal u = 66cm, and the angle against the diagonal is beta β = 57°43' - Nine-gon
Calculate the perimeter of a regular nonagon (9-gon) inscribed in a circle with a radius 18 cm. - High wall
I have a wall 2m high. I need a 15-degree angle (upward) to the second wall 4 meters away. How high must the second wall be? - Isosceles trapezoid
Isosceles trapezoid ABCD, AB||CD is given by |CD| = c = 12 cm, height v = 5 cm and |CAB| = 41°. Calculate area of the trapezoid. - IS triangle
Calculate the interior angles of the isosceles triangle with base 12 cm and legs 19 cm long. - Chord MN
Chord MN of the circle has distance from the center circle S 28 cm. Angle MSN is 54°. Determine the radius of the circle. - Overhangs 83158
The area of a right triangle ABC is 346 cm2, and the angle at vertex A is 64°. Calculate the lengths of the overhangs a and b. - Trapezoid 82216
Given is an isosceles trapezoid ABCD with bases 10 cm and 14 cm. The height of the trapezoid is 6 cm. Determine the interior angles of the trapezoid. - Trapezoids
In the isosceles trapezoid ABCD we know: AB||CD, |CD| = c = 8 cm, height h = 7 cm, |∠CAB| = 35°. Find the area of the trapezoid. - Circumscribed circle
In triangle ABC, we know a = 4 cm, b = 6 cm, γ = 60°. Calculate the area and radius of the inscribed and circumscribed circle. - Trapezoid 2520
Trapezoid with sides a = 10, b = 20, c = 25, d = 15. Calculate all internal angles.
Do you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
