Planimetrics - math word problems - page 160 of 184
Number of problems found: 3667
- Angles of elevation
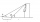From points A and B on level ground, the angles of elevation of the top of a building are 25° and 37°, respectively. If |AB| = 57m, calculate, to the nearest meter, the distances of the top of the building from A and B if they are both on the same side of - Right angle
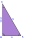In a right triangle ABC with a right angle at the apex C, we know the side length AB = 24 cm and the angle at the vertex B = 71°. Calculate the length of the legs of the triangle. - Calculate triangle
In the triangle, ABC, calculate the sizes of all heights, angles, perimeters, and areas if given a=40cm, b=57cm, and c=59cm. - A trapezoid
A trapezoid with a base length of a = 36.6 cm, with angles α = 60°, β = 48°, and the height of the trapezoid is 20 cm. Calculate the lengths of the other sides of the trapezoid. - Tree
How tall is the tree observed at the visual angle 45°? If I stand 3 m from the tree, my eyes are two meters above the ground. - Roof angle
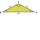The house's roof has the shape of an isosceles triangle with arms 4 m long and the size of the base 6 m. How big an angle alpha does its roof make? - How far
From the top of a lighthouse 145 ft above sea level, the angle of depression of a boat is 29°. How far is the boat from the lighthouse? - Square 81238
A forest with a square plan has an area of 4 square km. What side will the square have on a 1:50,000 scale map? - Elevation of the tower
We can see the top of the tower standing on a plane from a certain point A at an elevation angle of 39°25''. If we come towards its foot 50m closer to place B, we can see the top of the tower from it at an elevation angle of 56°42''. How tall is the tower - Depth angle
Determine the height of the cloud above the lake's surface if we see it from place A at an elevation angle of 20° 57'. From the same place A, we see its image in the lake at a depth angle of 24° 12'. Observation point A is 115 m above the lake level. - Difference 6469
The cable car rose at an angle of 15 °. The height difference between the upper and lower stations is 106m. Calculate the path's length. - Cable car 2
The cable car rises at an angle of 16° and connects the upper and lower station with an altitude difference of 1082 m. How long is the cable car's track? - The chimney
How high is the chimney if we see it from a distance of 60 m at an angle of 42°? - Tourist 39691
How far from the lookout tower, 48 m high, did the tourist stand if he saw its top at an angle of 40°? - The sequence
Find the nth term of the progression 2,6,12,20... - Right triangle
It is given a right triangle angle alpha of 90 degrees the beta angle of 55 degrees c = 10 cm use the Pythagorean theorem to calculate sides a and b - Two cables
On a flat plain, two columns are erected vertically upwards. One is 7 m high, and the other 4 m. Cables are stretched between the top of one column and the foot of the other column. At what height will the cables cross? Assume that the cables do not sag. - Flowerbed
The flowerbed has the shape of an obtuse isosceles triangle. The arm has a size of 5.5 meters, and an angle opposite the base size is 94°. What is the distance from the base to the opposite vertex? - Pentadecagon
Calculate the area of a regular 15-side polygon inscribed in a circle with a radius r = 4. Express the result to two decimal places. - Two chords
From the point on the circle with a diameter of 8 cm, two identical chords are led, which form an angle of 60°. Calculate the length of these chords.
Do you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
